how to find the difference quotient

What is the difference quotient in calculus? We start with the definition and then we calculate the difference quotient for different functions as examples with detailed explanations.

Definition of Difference Quotient

Let f be a function whose graph is shown below.

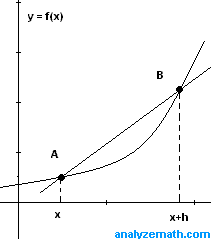
A and B are points on the graph of f. A line passing trough the two points A ( x , f(x)) and B(x+h , f(x+h)) is called a secant line. The slope m of the secant line may be calculated as follows:

m = \dfrac{f (x + h) - f(x)}{(x + h) - x}

Simplify the denominator to obtain

m = \dfrac{f (x + h) - f(x)}{h}

This slope is very important in calculus where it is used to define the derivative of function f which in fact defines the local variation of a function in mathematics. It is called the difference quotient . In the examples below, we calculate and simplify the difference quotients of different functions.

Example 1

Find the difference quotient of function f defined by

f(x) = 2x + 5

Solution to Example 1

We first need to calculate f(x + h).
f(x + h) = 2(x + h) + 5
We now substitute f(x + h) and f(x) in the definition of the difference quotient by their expressions
\dfrac{f (x + h) - f(x)}{h} = \dfrac{2(x + h) + 5 - (2 x + 5) }{h}

We simplify the above expression.
= \dfrac{2h}{2} = 2
The answer is 2 which also the slope of the graph of function f, why?

Example 2

Find the difference quotient of the following function

f(x) = 2x² + x - 2

Solution to Example 2

We first calculate f(x + h).
f(x + h) = 2(x + h)^2 + (x + h) - 2
We now substitute f(x + h) and f(x) in the difference quotient
\dfrac{f (x + h) - f(x)}{h} = \dfrac{ 2(x + h)^2 + (x + h) - 2 - ( 2 x^2 + x - 2 )}{h}
We expand the expressions in the numerator and group like terms.
= \dfrac{ 4 x h + 2 h^2 + h}{h} = 4 x + 2 h +1

Example 3

Find the difference quotient of function f given by

f(x) = sin x

Solution to Example 3

We first calculate f(x + h).
f(x + h) = \sin (x + h)
We now substitute f(x + h) and f(x) in the difference quotient
\dfrac{f (x + h) - f(x)}{h} = \dfrac{ \sin (x + h) - \sin x}{h}
We use the trigonometric formula that transform a difference sin (x + h) - sin x into a product.
\sin (x + h) - \sin x = 2 \cos [ (2 x + h)/2 ] \sin (h/2)
We substitute the above expression for sin (x + h) - sin x in the difference quotient above to obtain.
\dfrac{f (x + h) - f(x)}{h} = \dfrac{ 2 \cos [ (2 x + h)/2 ] \sin (h/2)}{h}